|
|
|
|
|
Next: 6.19 DOS for a Periodic Box |
|
The single-particle quantum states, or energy eigenfunctions, for
noninteracting particles in a closed box were given in section
6.2, (6.2). They were a product of a sine in
each axial direction. Those for a periodic box can similarly be taken
to be a product of a sine or cosine in each direction. However, it is
usually much better to take the single-particle energy eigenfunctions
to be exponentials:
One major advantage of these eigenfunctions is that they are also
eigenfunction of linear momentum. For example. the linear momentum in
the ![]() -
-![]()
![]()
![]() .
.![]() -
-![]()
![]()
![]()
The reason that the momentum eigenfunctions are also energy eigenfunctions is that the energy is all kinetic energy. It makes the energy proportional to the square of linear momentum. (The same is true inside the closed box, but momentum eigenstates are not acceptable states for the closed box. You can think of the surfaces of the closed box as infinitely high potential energy barriers. They reflect the particles and the energy eigenfunctions then must be a 50/50 mix of forward and backward momentum.)
Like for the closed box, for the periodic box the single-particle
energy is still given by
Unlike for the closed box however, the wave numbers ![]() ,
,![]() ,
,![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.![]()
![]() ,
,
In addition, unlike for the sinusoidal eigenfunctions of the closed
box, zero and negative values of the wave numbers must now be allowed.
Otherwise the set of eigenfunctions will not be complete. The
difference is that for the closed box, ![]()
![]() ,
,![]()
![]()
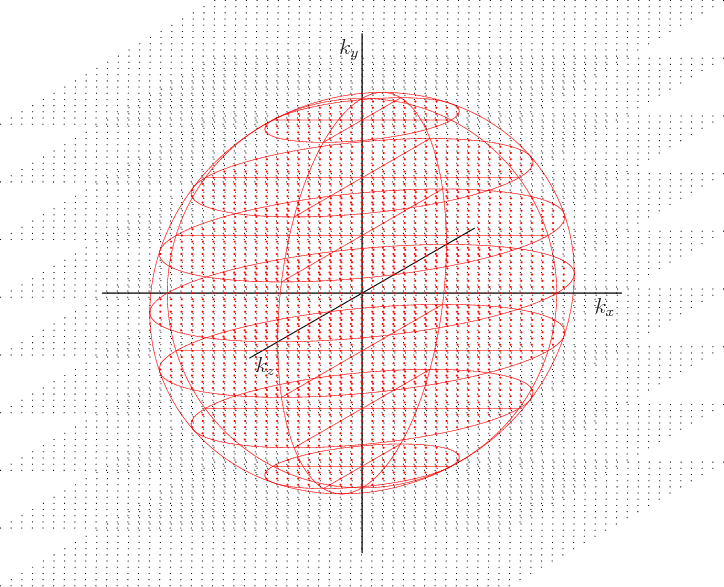 |
Figure 6.17 shows the wave number space for a system of electrons in a periodic box. The wave number vectors are no longer restricted to the first quadrant like for the closed box in figure 6.11; they now fill the entire space. In the ground state, the states occupied by electrons, shown in red, now form a complete sphere. For the closed box they formed just an octant of one. The Fermi surface, the surface of the sphere, is now a complete spherical surface.
It may also be noted that in later parts of this book, often the wave
number vector or momentum vector is used to label the eigenfunctions:
Key Points
- The energy eigenfunctions for a periodic box are usually best taken to be exponentials. Then the wave number values can be both positive and negative.
- The single-particle kinetic energy is still
.
- The momentum is
.
- The eigenfunction labelling may vary.