|
|
|
|
|
Next: 13.4 Particles in Magnetic Fields |
|
In this section, some basic solutions of Maxwell’s equations are described. They will be of interest in addendum {A.39} for understanding relativistic effects on the hydrogen atom (though certainly not essential). They are also of considerable practical importance for a lot of nonquantum applications.
It is assumed throughout this subsection that the electric and magnetic fields do not change with time. All solutions also assume that the ambient medium is vacuum.
For easy reference, Maxwell’s equations and various results to be
obtained in this section are collected together in tables
13.1 and 13.2. While the existence of
magnetic monopoles is unverified, it is often convenient to compute as
if they do exist. It allows you to apply ideas from the electric
field to the magnetic field and vice-versa. So, the tables include
magnetic monopoles with strength ![]() ,
,![]() ,
,![]()
![]() .
.![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
![]()
A point charge is a charge concentrated at a single point. It is a
very good model for the electric field of the nucleus of an atom,
since the nucleus is so small compared to the atom. A point charge of
strength ![]()
| (13.13) |
The electric field lines of a point charge are radially outward from
the charge; see for example figure 13.3 in the previous
subsection. According to Coulomb’s law, the electric field of a
point charge is
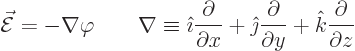
voltage.It follows by integration of the electric field strength with respect to
Delta functions are often not that easy to work with analytically,
since they are infinite and infinity is a tricky mathematical thing.
It is often easier to do the mathematics by assuming that the charge
is spread out over a small sphere of radius ![]() ,
,![]() ,
,
 |
(13.16) |
![\begin{figure}\centering
\setlength{\unitlength}{1pt}
\begin{picture}(360,11...
...cal E}$}}
\put(300,-3){\makebox(0,0)[b]{$\varphi$}}
\end{picture}
\end{figure}](img2866.gif) |
Figure 13.7 shows that outside the region with charge, the
electric field and potential are exactly like those of a point charge
with the same net charge ![]() .
.![]()
![]()
![]()
If magnetic monopoles exist, they would create a magnetic field much
like an electric charge creates an electric field. As table
13.1 shows, the only difference is the square of the
speed of light ![]()
A homogeneous distribution of charges along an infinite straight line
is called a line charge. As shown in figure 13.8, it
creates a two-dimensional field in the planes normal to the line. The line
charge becomes a point charge within such a plane. The expression for
the field of a line charge can be derived in much the same way as
Coulomb’s law was derived for a three-dimensional point charge in the
previous section. In particular, where that derivation surrounded the
point charge by a spherical surface, surround the line charge by a
cylinder. (Or by a circle, if you want to think of it in two
dimensions.) The resulting expressions are given in table
13.1; they are in terms of the charge per unit length of
the line ![]() .
.
A point charge can describe a single charged particle like an atom
nucleus or electron. But much of the time in physics, you are dealing
with neutral atoms or molecules. For those, the net charge is zero.
The simplest model for a system with zero net charge is called the
dipole.
It is simply a combination of a positive point
charge ![]()
![]()
![]() ,
,
Figure 13.9 shows an example of a dipole in which the
positive charge is straight above the negative one. Note the
distinctive egg shape of the biggest electric field lines. The
electric dipole moment
![]()
![]()
The potential of a dipole is simply the sum of the potentials of the
two charges:
The electric field of the dipole can be found from either taking minus
the gradient of the potential above, or from adding the fields of the
individual point charges, and is
To obtain that result from taking the the gradient of the potential,
remember the following important formula for the gradient of
![]()
![]()
Similar expressions apply for magnetic dipoles. The field outside a thin bar magnet can be approximated as a magnetic dipole, with the north and south poles of the magnet as the positive and negative magnetic point charges. The magnetic field lines are then just like the electric field lines in figure 13.9.
Corresponding expressions can also be written down in two dimensions, for opposite charges distributed along parallel straight lines. Figure 13.10 gives an example. In two dimensions, all field lines are circles passing through both charges.
A particle like an electron has an electric charge and no known size.
It can therefore be described as an ideal point charge. But an
electron also has a magnetic moment: it acts as a magnet of zero size.
Such a magnet of zero size will be referred to as an “ideal magnetic dipole.” More precisely, an ideal
magnetic dipole is defined as the limit of a magnetic dipole when the
two poles are brought vanishingly close together. Now if you just let
the two poles approach each other without doing anything else, their
opposite fields will begin to increasingly cancel each other, and
there will be no field left when the poles are on top of each other.
When you make the distance between the poles smaller, you also need to
increase the strengths ![]()
| (13.21) |
The field lines of a vertical ideal magnetic dipole are shown in
figure 13.11. Their egg shape is in spherical coordinates
described by, {D.72},
![\begin{displaymath}
\varphi_m = \frac{q_m}{4\pi\epsilon_0c^2} \left[
\frac{1}{...
...1}{\vert{\skew0\vec r}-{\skew0\vec r}_{\ominus}\vert} \right]
\end{displaymath}](img2884.gif)
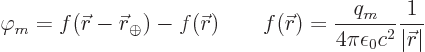
Similar expressions can be written down for ideal electric dipoles and in two-dimensions. They are listed in tables 13.1 and 13.2. (The delta functions will be discussed in the next subsection.)
Figure 13.12 shows an almost ideal two-dimensional electric dipole. The spacing between the charges has been reduced significantly compared to that in figure 13.10, and the strength of the charges has been increased. For two-dimensional ideal dipoles, the field lines in a cross-plane are circles that all touch each other at the dipole.
Modeling electric systems like atoms and molecules and their ions as
singular point charges or dipoles is not very accurate, except in a
detailed quantum solution. In a classical description, it is more
reasonable to assume that the charges are smeared out
over space into a distribution. In that case, the charges are
described by the charge per unit volume, called the charge density
![]() .
.
 |
(13.25) |
As far as the potential is concerned, each little piece
![]()
![]() .
.![]()
![]() .
.
Note that when the integral expression for the potential is
differentiated to find the electric field, as in table
13.2, the integrand becomes much more singular at the
point of integration where ![]()
![]()
![]() .
.
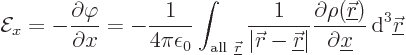
Now consider the case that the charge distribution is restricted to a
very small region around the origin, or equivalently, that the charge
distribution is viewed from a very large distance. For simplicity,
assume the case that the charge distribution is restricted to a small
region around the origin. In that case, ![]()
![]()
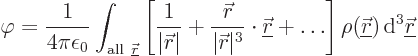
Since the fractions no longer involve ![]() ,
,
The expansion (13.27) is called a “multipole expansion.” It allows the effect of a complicated charge distribution to be described by a few simple terms, assuming that the distance from the charge distribution is sufficiently large that its small scale features can be ignored. If necessary, the accuracy of the expansion can be improved by using more terms in the Taylor series. Now recall from the previous section that one advantage of Maxwell’s equations over Coulomb’s law is that they allow you to describe the electric field at a point using purely local quantities, rather than having to consider the charges everywhere. But using a multipole expansion, you can simplify the effects of distant charge distributions. Then Coulomb’s law can become competitive with Maxwell’s equations, especially in cases where the charge distribution is restricted to a relatively limited fraction of the total space.
The previous subsection discussed how an ideal dipole could be created
by decreasing the distance between two opposite charges with a
compensating increase in their strength. The multipole expansion
above shows that the same ideal dipole is obtained for a continuous
charge distribution, provided that the net charge ![]()
The electric field of this ideal dipole can be found as minus the
gradient of the potential. But caution is needed; the so-obtained
electric field may not be sufficient for your needs. Consider the
following ballpark estimates. Assume that the charge distribution has
been contracted to a typical small size ![]() .
.![]() .
.![]() ,
,![]() ,
,![]() .
.![]()
![]()
![]() .
.
The previous subsections stumbled onto the solution of an important
mathematical problem, the Poisson equation. The Poisson equation is
| (13.28) |
The reason that the previous subsection stumbled on to the solution of
this equation is that the electric potential ![]()
![]()
![]()
![]() .
.![]()
![]()
![]()
![]()
![]() .
.![]()
![]()
![]()
![]() ,
,
Because it is such an important problem, it is a good idea to write
out the abstract mathematical solution without the “physical
entourage” of (13.26):
It also follows that applying the Laplacian on the Green’s
function produces the three-dimensional delta function,
The multipole expansion for a charge distribution can also be
converted to purely mathematical terms:
(Of course, delta functions are infinite objects, and you might wonder at the mathematical rigor of the various arguments above. However, there are solid arguments based on “Green’s second integral identity” that avoid the infinities and produce the same final results.)
Streams of moving electric charges are called currents. The current
strength ![]()
![]()
![]() ;
;
As shown in figure 13.13, electric wires are encircled by
magnetic field lines. The strength of this magnetic field may be
computed from Maxwell’s fourth equation. To do so, take an
arbitrary field line circle. The field strength is constant on the
line by symmetry. So the integral of the field strength along the
line is just ![]() ;
;![]()
![]()
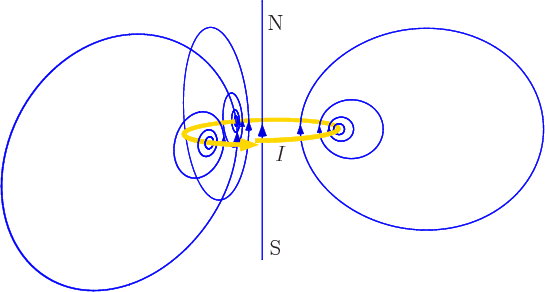 |
An infinite straight wire is of course not a practical way to create a magnetic field. In a typical electromagnet, the wire is spooled around an iron bar. Figure 13.14 shows the field produced by a single wire loop, in vacuum. To find the fields produced by curved wires, use the so-called “Biot-Savart law” listed in table 13.2 and derived in {D.72}. You need it when you end up writing a book on quantum mechanics and have to plot the field.
Of course, while figure 13.14 does not show it, you will
also need a lead from your battery to the electromagnet and a second
lead back to the other pole of the battery. These two leads form a
two-dimensional current dipole,
as shown in figure
13.15, and they produce a magnetic field too. However, the
currents in the two leads are opposite; one coming from the battery
and other returning to it, so the magnetic fields that they create are
opposite. Therefore, if you strand the wires very closely together,
their magnetic fields will cancel each other, and not mess up that of
your electromagnet.
It may be noted that if you bring the wires close together, whatever is left of the field has circular field lines that touch at the dipole. In other words, a horizontal ideal current dipole produces the same field as a two-dimensional vertical ideal charge dipole. Similarly, the horizontal wire loop, if small enough, produces the same field lines as a three-dimensional vertical ideal charge dipole. (However, the delta functions are different, {D.72}.)
The previous section discussed how Maxwell’s third equation allows electric power generation using mechanical means. The converse is also possible; electric power allows mechanical power to be generated; that is the principle of the electric motor.
![\begin{figure}\centering
\setlength{\unitlength}{1pt}
\begin{picture}(300,18...
...}_{\rm ext}$}}
\put(-124,56){\makebox(0,0)[b]{$M$}}
\end{picture}
\end{figure}](img2920.gif) |
It is possible because of the Lorentz force law, which says that a
charge ![]()
![]()
![]()
![\begin{figure}\centering
\setlength{\unitlength}{1pt}
\begin{picture}(300,18...
...0)[b]{$\phi$}}
\put(-124,56){\makebox(0,0)[b]{$M$}}
\end{picture}
\end{figure}](img2923.gif) |
To be more precise, the forces caused by the component of the magnetic
field normal to the wire loop are radial and produce no net force nor
moment. However, the forces caused by the component of the magnetic
field parallel to the loop produce forces normal to the plane of the
loop that do generate a net moment. Using spherical coordinates
aligned with the wire loop as in figure 13.17, the
component of the magnetic field parallel to the loop equals
![]() .
.![]()
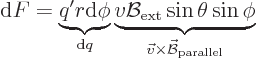
The magnetic dipole moment ![]()
![]()
![]()
![]()
A book on electromagnetics would typically identify ![]()
![]()
![]()
![]() .
.
But this is a book on quantum mechanics, and for electrons in orbits
about nuclei, currents and areas are not very useful. In quantum
mechanics the more meaningful quantity is angular momentum. So
identify ![]()
![]()
![]()
![]()
![]()
![]() .
.
These results apply to any arbitrary current distribution, not just a circular wire loop. Formulae are in table 13.2 and general derivations in {D.72}.