D.68 Awk­ward ques­tions about spin

Now of course you ask: how do you know how the math­e­mat­i­cal ex­pres­sions for spin states change when the co­or­di­nate sys­tem is ro­tated around some axis? Darn.

If you did a ba­sic course in lin­ear al­ge­bra, they will have told you how the com­po­nents of nor­mal vec­tors change when the co­or­di­nate sys­tem is ro­tated, but not spin vec­tors, or spin­ors, which are two-di­men­sion­al vec­tors in three-di­men­sion­al space.

You need to go back to the fun­da­men­tal mean­ing of an­gu­lar mo­men­tum. The ef­fect of ro­ta­tions of the co­or­di­nate sys­tem around the $z$-​axis was dis­cussed in ad­den­dum {A.19}. The ex­pres­sions given there can be straight­for­wardly gen­er­al­ized to ro­ta­tions around a line in the di­rec­tion of an ar­bi­trary unit vec­tor $(n_x,n_y,n_z)$. Ro­ta­tion by an an­gle $\varphi$ mul­ti­plies the $n$-​di­rec­tion an­gu­lar mo­men­tum eigen­states by $e^{{\rm i}{m}\varphi}$ if $m\hbar$ is the an­gu­lar mo­men­tum in the $n$-​di­rec­tion. For elec­tron spin, the val­ues for $m$ are $\pm\frac12$, so, us­ing the Euler for­mula (2.5) for the ex­po­nen­tial, the eigen­states change by a fac­tor

$$\cos\left({\textstyle\frac{1}{2}}\varphi\right) \pm {\rm i}\sin\left({\textstyle\frac{1}{2}}\varphi\right)$$

For ar­bi­trary com­bi­na­tions of the eigen­states, the first of the two terms above still rep­re­sents mul­ti­pli­ca­tion by the num­ber $\cos\left(\frac12\varphi\right)$.

The sec­ond term may be com­pared to the ef­fect of the $n$-​di­rec­tion an­gu­lar mo­men­tum op­er­a­tor ${\widehat J}_n$, which mul­ti­plies the an­gu­lar mo­men­tum eigen­states by $\pm\frac12\hbar$; it is seen to be $2{\rm i}\sin\left({\textstyle\frac{1}{2}}\varphi\right){\widehat J}_n$$\raisebox{.5pt}{$/$}$​$\hbar$. So the op­er­a­tor that de­scribes ro­ta­tion of the co­or­di­nate sys­tem over an an­gle $\varphi$ around the $n$-​axis is

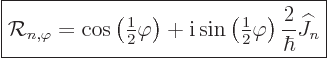$$\fbox{$\displaystyle {\cal R}_{n,\varphi} = \cos\left({\t... ...le\frac{1}{2}}\varphi\right) \frac{2}{\hbar}{\widehat J}_n $}$$ (D.44)

Fur­ther, in terms of the $x$, $y$, and $z$ an­gu­lar mo­men­tum op­er­a­tors, the an­gu­lar mo­men­tum in the $n$-​di­rec­tion is

$${\widehat J}_n = n_x {\widehat J}_x + n_y {\widehat J}_y + n_z {\widehat J}_z$$

If you put it in terms of the Pauli spin ma­tri­ces, $\hbar$ drops out:

$${\cal R}_{n,\varphi} = \cos\left({\textstyle\frac{1}{2}}\v... ...ight) \left(n_x \sigma_x + n_y \sigma_y + n_z \sigma_z\right)$$

Us­ing this op­er­a­tor, you can find out how the spin-up and spin-down states are de­scribed in terms of cor­re­spond­ingly de­fined ba­sis states along the $x$-​ or $y$-​axis, and then de­duce these cor­re­spond­ingly de­fined ba­sis states in terms of the $z$ ones.

Note how­ever that the very idea of defin­ing the pos­i­tive $x$ and $y$ an­gu­lar mo­men­tum states from the $z$ ones by ro­tat­ing the co­or­di­nate sys­tem over 90$\POW9,{\circ}$ is some­what spe­cious. If you ro­tate the co­or­di­nate sys­tem over 450$\POW9,{\circ}$ in­stead, you get a dif­fer­ent an­swer! Off by a fac­tor $\vphantom{0}\raisebox{1.5pt}{$-$}$1, to be pre­cise. But that is as bad as the in­de­ter­mi­nacy gets; what­ever way you ro­tate the axis sys­tem to the new po­si­tion, the ba­sis vec­tors you get will ei­ther be the same or only a fac­tor $\vphantom{0}\raisebox{1.5pt}{$-$}$1 dif­fer­ent {D.69}.

More awk­wardly, the neg­a­tive mo­men­tum states ob­tained by ro­ta­tion do not lead to real pos­i­tive nu­mer­i­cal fac­tors for the cor­re­spond­ing lad­der op­er­a­tors. Pre­sum­ably, this re­flects the fact that at the wave func­tion level, na­ture does not have the ro­ta­tional sym­me­try that it has for ob­serv­able quan­ti­ties. Any­way, if na­ture does not bother to obey such sym­me­try, then there seems no point in pre­tend­ing it does. Es­pe­cially since the non­pos­i­tive lad­der fac­tors would mess up var­i­ous for­mu­lae. The neg­a­tive spin states found by ro­ta­tion go out of the win­dow. Bye, bye.