|
|
|
|
|
Next: 6.11 Degeneracy Pressure |
|
As the previous section discussed, a system of noninteracting electrons, a free-electron gas, occupies a range of single-particle energies. Now the electrons with the highest single-particle energies are particularly important. The reason is that these electrons have empty single-particle states available at just very slightly higher energy. Therefore, these electrons are easily excited to do useful things, like conduct electricity for example. In contrast, electrons in energy states of lower energy do not have empty states within easy reach. Therefore lower energy electron are essentially stuck in their states; they do not usually contribute to nontrivial electronic effects.
Valence electrons in metals behave qualitatively much like a
free-electron gas. For them too, the electrons in the highest energy
single-particle states are the critical ones for the metallic
properties. Therefore, the highest single-particle energy occupied by
electrons in the system ground state has been given a special name;
the Fermi energy.
In the energy spectrum of the
free-electron gas to the right in figure 6.11, the Fermi
energy is indicated by a red tick mark on the axis.
Also, the surface that the electrons of highest energy occupy in wave
number space is called the Fermi surface.
For the
free-electron gas the wave number space was illustrated to the left
in figure 6.11. The Fermi surface is outlined in red in the
figure; it is the spherical outside surface of the occupied region.
One issue that is important for understanding the properties of systems
of electrons is the overall magnitude of the Fermi energy. Recall
first that for a system of bosons, in the ground state all bosons are
in the single-particle state of lowest energy. That state corresponds
to the point closest to the origin in wave number space. It has very
little energy, even in terms of atomic units of electronic energy.
That was illustrated numerically in table 6.1. The
lowest single-particle energy is, assuming that the box is cubic
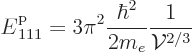 |
(6.15) |
Unlike for bosons, for electrons only two electrons can go into the
lowest energy state. Or in any other spatial state for that matter.
And since a macroscopic system has a gigantic number of electrons, it
follows that a gigantic number of states must be occupied in wave
number space. Therefore the states on the Fermi surface in figure
6.11 are many orders of magnitude further away from the
origin than the state of lowest energy. And since the energy is
proportional to the square distance from the origin, that means that
the Fermi energy is many orders of magnitude larger than the lowest
single-particle energy ![]() .
.
More precisely, the Fermi energy of a free-electron gas can be
expressed in terms of the number of electrons per unit volume ![]()
![]()
![]()
It follows that the Fermi energy is larger than the lowest
single-particle energy by the gigantic factor
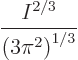
It is instructive to put some ballpark number to the Fermi energy. In
particular, take the valence electrons in a block of copper as a
model. Assuming one valence electron per atom, the electron density
![]()
![]()
![]()
![]()
![]()
![]() ,
,![]()
The Fermi energy gives the maximum energy that an electron can have.
The average energy that they have is comparable but somewhat smaller:
| (6.17) |
For copper, the ballpark average energy is 4.2 eV. To put that in
context, consider the equivalent temperature at which classical
particles would need to be to have the same average kinetic energy.
Multiplying 4.2 eV by ![]()
![]()
![]()
Key Points
- The Fermi energy is the highest single-particle energy that a system of electrons at absolute zero temperature will occupy.
- It is normally a very high energy.
- The Fermi surface is the surface that the electrons with the Fermi energy occupy in wave number space.
- The average energy per electron for a free-electron gas is 60% of the Fermi energy.