|
|
|
|
|
Next: 8.3 Global Symmetrization |
|
Special relativity has shown that we humans cannot transmit information at more than the speed of light. However, according to the orthodox interpretation, nature does not limit itself to the same silly restrictions that it puts on us. This section discusses why not.
Consider again the ![]() -
-
You might send one proton off to your observer on Mars, the other to your observer on Venus. Where is the electron, on Mars or on Venus?
According to the orthodox interpretation, the answer is: neither. A position for the electron does not exist. The electron is not on Mars. It is not on Venus. Only when either observer makes a measurement to see whether the electron is there, nature throws its dice, and based on the result, might put the electron on Venus and zero the wave function on Mars. But regardless of the distance, it could just as well have put the electron on Mars, if the dice would have come up differently.
You might think that nature cheats, that when you take the protons apart, nature already decides where the electron is going to be. That the Venus proton secretly hides the electron “in its sleeve”, ready to make it appear if an observation is made. John Bell devised a clever test to force nature to reveal whether it has something hidden in its sleeve during a similar sort of trick.
The test case Bell used was a generalization of an experiment proposed
by Bohm. It involves spin measurements on an electron/positron pair,
created by the decay of an ![]() -
-
Now suppose Earth happens to be almost the same distance from Mars and Venus, and you shoot the positron out to Venus, and the electron to Mars, as shown at the left in the figure below:
![\begin{figure}\centering
\setlength{\unitlength}{1pt}
% -125 left aligns the...
...b]{Earth}}
\put(70.7,17){\makebox(0,0)[b]{Mars}}
}
\end{picture}
\end{figure}](img1906.gif) |
You have observers on both planets waiting for the particles. According to quantum mechanics, the traveling electron and positron are both in an indeterminate state.
The positron reaches Venus a fraction of a second earlier, and the observer there measures its spin in the direction up from the ecliptic plane. According to the orthodox interpretation, nature now makes a random selection between the two possibilities, and assume it selects the positive spin value for the positron, corresponding to a spin that is up from the ecliptic plane, as shown in figure 8.2. Immediately, then, the spin state of the electron on Mars must also have collapsed; the observer on Mars is guaranteed to now measure negative spin, or spin down, for the electron.
The funny thing is, if you believe the orthodox interpretation, the information about the measurement of the positron has to reach the electron instantaneously, much faster than light can travel. This apparent problem in the orthodox interpretation was discovered by Einstein, Podolski, and Rosen. They doubted it could be true, and argued that it indicated that something must be missing in quantum mechanics.
In fact, instead of superluminal effects, it seems much more
reasonable to assume that earlier on earth, when the particles were
sent on their way, nature attached a secret little
note
of some kind to the positron, saying the
equivalent of “If your spin up is measured, give the positive
value”, and that it attached a little note to the electron
If your spin up is measured, give the negative value.
The results of the measurements are still the same, and the little
notes travel along with the particles, well below the speed of light,
so all seems now fine. Of course, these would not be true notes, but
some kind of additional information beyond the normal quantum
mechanics. Such postulated additional information sources are
called “hidden variables.”
Bell saw that there was a fundamental flaw in this idea if you do a
large number of such measurements and you allow the observers to
select from more than one measurement direction at random. He derived
a neat little general formula, but the discussion here will just show
the contradiction in a single case. In particular, the observers on
Venus and Mars will be allowed to select randomly one of three
measurement directions ![]() ,
,![]() ,
,![]()
Let’s see what the little notes attached to the electrons might
say. They might say, for example, “Give the ![]()
![]()
![]()
![]()
![]()
![]()
![]() .
.
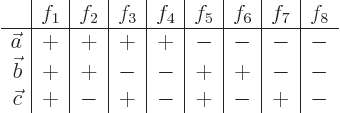
Of course, each note attached to the positron must always be just the
opposite of the one attached to the electron, since the positron must
measure ![]()
![]()
Now consider those measurements in which the Venus observer measures
direction ![]()
![]() .
.![]() .
.![]()
![]() .
.![]()
![]()
![]() ”
”![]()
![]() ,
,![]() ”
”![]() a
a![]()
![]()
![]()
![]() :
:![]() .
.
Similarly, the fraction of cases where Venus finds ![]()
![]() i
i![]() ,
,
A seemingly perfectly logical and plausible explanation by great minds is tripped up by some numbers that just do not want to match up. They only leave the alternative nobody really wanted to believe.
Attaching notes does not work. Information on what the observer on Venus decided to measure, the one thing that could not be put in the notes, must have been communicated instantly to the electron on Mars regardless of the distance.
It can also safely be concluded that we humans will never be able to see inside the actual machinery of quantum mechanics. For, suppose the observer on Mars could see the wave function of the electron collapse. Then the observer on Venus could send her Morse signals faster than the speed of light by either measuring or not measuring the spin of the positron. Special relativity would then allow signals to be sent into the past, and that leads to logical contradictions such as the Venus observer preventing her mother from having her.
While the results of the spin measurements can be observed, they do not allow superluminal communication. While the observer on Venus affects the results of the measurements of the observer on Mars, they will look completely random to that observer. Only when the observer on Venus sends over the results of her measurements, at a speed less than the speed of light, and the two sets of results are compared, do meaningful patterns how up.
The Bell experiments are often used to argue that Nature must really make the collapse decision using a true random number generator, but that is of course crap. The experiments indicate that Nature instantaneously transmits the collapse decision on Venus to Mars, but say nothing about how that decision was reached.
Superluminal effects still cause paradoxes, of course. The left of figure 8.4 shows how a Bohm experiment appears to an observer on earth.
The spins remain undecided until the measurement by the Venus observer causes both the positron and the electron spins to collapse.
However, for a moving observer, things would look very different. Assuming that the observer and the particles are all moving at speeds comparable to the speed of light, the same situation may look like the right of figure 8.4, chapter 1.1.4. In this case, the observer on Mars causes the wave function to collapse at a time that the positron has only just started moving towards Venus!
So the orthodox interpretation is not quite accurate. It should really have said that the measurement on Venus causes a convergence of the wave function, not an absolute collapse. What the observer of Venus really achieves in the orthodox interpretation is that after her measurement, all observers agree that the positron wave function is collapsed. Before that time, some observers are perfectly correct in saying that the wave function is already collapsed, and that the Mars observer did it.
It should be noted that when the equations of quantum mechanics are correctly applied, the collapse and superluminal effects disappear. That is explained in section 8.6. But, due to the fact that there are limits to our observational capabilities, as far as our own human experiences are concerned, the paradoxes remain real.
To be perfectly honest, it should be noted that the example above is
not quite the one of Bell. Bell really used the inequality: