| Quantum Mechanics Solution Manual |
|
© Leon van Dommelen |
|
4.3.3.2 Solution hydc-b
Question:
What is the value of energy level 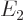 ? And
? And  ?
?
Answer:
No need to put all the numbers into
because the only difference between 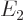 and
and  is just a final factor
is just a final factor  . So
. So 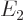



 3.4 eV. Similarly,
3.4 eV. Similarly, 



 1.51 eV.
1.51 eV.
![]() ?
?![]() ?
?