| Quantum Mechanics Solution Manual |
|
© Leon van Dommelen |
|
5.5.2.1 Solution complexsai-a
Question:
Show that the normalization requirement for the wave function of a spin 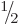 particle in terms of
particle in terms of 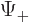 and
and 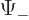 requires its norm
requires its norm 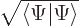 to be one.
to be one.
Answer:
As a corresponding question in the previous subsection discussed; the total probability of finding the particle somewhere with spin up is 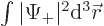 , and the total probability of finding it somewhere with spin down is
, and the total probability of finding it somewhere with spin down is 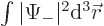 . The sum of the two integrals must be one to express the fact that the probability of finding the particle somewhere, either with spin up or spin down, must be one, certainty.
. The sum of the two integrals must be one to express the fact that the probability of finding the particle somewhere, either with spin up or spin down, must be one, certainty.
Compare that with the square norm of the wave function, which is by definition the inner product of the wave function with itself:
and the final two inner products are by definition the two integrals above. Since their sum must be one, it follows that the norm of the wave function 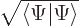 must be one even if there is spin.
must be one even if there is spin.
![]()
![]()
![]()
![]()
![]() ,
,![]() .
.