6 Cycle Devices

Exams 3 and final only

For cycle devices, the normal sign conventions (heat in and work out are positive) are ignored. Instead, the signs of heat and work are defined such that $\dot Q_H$ , $\dot Q_L$ and net work $\dot W$ are normally positive. The pictures below show the directions of positive heat fluxes and work rates for a heat engine as compared to a heat pump (or refrigeration cycle).

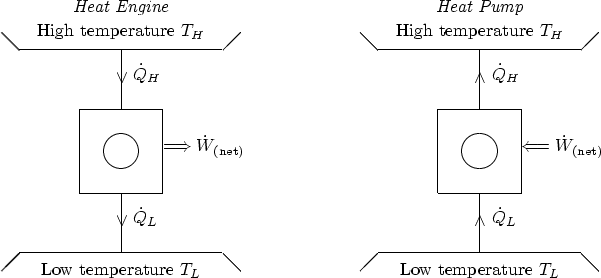
$\begin{picture}(1392,460)(0,0) \par \put(196,0){ \begin{picture}(400,460)(0,0) ... ...put(200,0){\makebox(0,0){Low temperature $T_L$}} \end{picture} } \end{picture}$

The first law for a cycle:

$\begin{displaymath} \mbox{Net work } {\mbox{out} \atop \mbox{in}} = \mbox{Ne... ...t}}\mbox{:} \qquad W_{\mbox{\scriptsize (net)}} = Q_H - Q_L \end{displaymath}$

The Kelvin-Planck Statement: Any working heat engine must dump some waste heat to a lower temperature than the input heat is provided at.

The Clausius Statement: Any working heat pump must have positive work going in.

Thermal efficiency:

$\begin{displaymath} \eta_{\mbox{\scriptsize th}} \equiv \frac{\dot W_{\mbox{... ...d \eta_{\mbox{\scriptsize th, rev}} = \frac{T_H-T_L}{T_H} \end{displaymath}$

Refrigeration coefficient of performance:

$\begin{displaymath} {\rm COP}_{\rm R} \equiv \frac{\dot Q_L}{\dot W_{\mbox{\... ...\quad\le\quad {\rm COP}_{\rm R,rev} = \frac{T_L}{T_H-T_L} \end{displaymath}$

Heating heat pump coefficient of performance $\beta' = 1 + \beta$ :

$\begin{displaymath} {\rm COP}_{\rm HP} \equiv \frac{\dot Q_H}{\dot W_{\mbox{... ...quad\le\quad {\rm COP}_{\rm HP,rev} = \frac{T_H}{T_H-T_L} \end{displaymath}$

Ideal gas Carnot cycle:

$\begin{displaymath} q_H = q_{12} = R T_H \ln\left(\frac{v_2}{v_1}\right) \qq... ...v_3}{v_4}\right) \qquad \frac{v_2}{v_1} = \frac{v_3}{v_4} \end{displaymath}$

End exams 3 and final only