Next: Smoothness of the
Up: The heat equation.
Previous: The initial condition.
What truly makes the PDE different from the initial and boundary
conditions is the region of applicability. The PDE applies at all
points. (At least, if we ignore the possibility that some points could
be singular). The initial and boundary conditions only apply at a
small subset of points in the x,t-plane of figure 2.
It is the PDE that must be integrated into the field. You cannot,
say, take the Neumann boundary condition into the interior. Clearly,
it is in general not true that 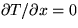 in the
interior.
in the
interior.
Next: Smoothness of the
Up: The heat equation.
Previous: The initial condition.