| Quantum Mechanics Solution Manual |
|
© Leon van Dommelen |
|
2.4.3 Solution mathops-c
Question:
A less self-evident operator than the above examples is a translation operator like  that translates the graph of a function towards the left by an amount
that translates the graph of a function towards the left by an amount 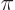
 2:
2: 

 . (Curiously enough, translation operators turn out to be responsible for the law of conservation of momentum.) Show that
. (Curiously enough, translation operators turn out to be responsible for the law of conservation of momentum.) Show that  turns
turns  into
into  .
.
Answer:
Using various standard trig manipulations, [1, pp. 43-44]:
Or just compare the graphs visually, [1, p. 43].
![]()
![]()
![]()
![]()
![]()
![]() .
.![]()
![]()
![]() .
.