EML 5060 Analysis in Mechanical Engineering 10/22/99
Closed book Van Dommelen 10:45-11:35am

Show all reasoning and intermediate results leading to your answer, or credit will be lost. One book of mathematical tables, such as Schaum's Mathematical Handbook, may be used, as well as a calculator.

1.

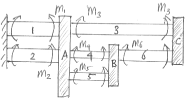
The construction below consists of beams 1 through 6 and slabs A, B, and C welded together as shown. Beams 1 and 2 are welded to a fixed wall. Due to construction tolerances, there are internal bending moments M₁ through M₆ in the bars. Write equilibrium equations for slabs A, B and C. Reduce this system to echelon form and thus find a basis to the null space. From it, show that the internal moment field is always a superposition of a small number of independent simple moment fields. Identify and describe these independent simple internal fields. Solution.

2.

A point spirals along the surface of the cylinder of radius a around the z-axis according to

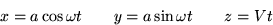
where a, 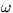, and V are given constants. Find the tangential unit vector to the path as well as the arclength travelled per unit time. Also find the unit vector pointing from the point to the center of curvature of the path. Find the radius of curvature of the path and show that it is greater than the radius a of the cylinder along which the point is moving. Solution.

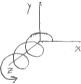
3.

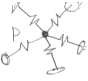
The point below is suspended by a variety of springs. The potential energy stored in the springs when the point moves away from the origin is:

U_p = 5 x² + 5 y² + z² + 2 xz + 4 yz

To what principal form can this be simplified by a suitable rotation of the coordinate system? From this simplified expression, show that the springs do not oppose motion in a certain direction. Give the unit vector in that direction. Solution.