


Up: Return
EML 5060 Analysis in Mechanical Engineering 9/16/98
Closed book Van Dommelen 11:00-11:50am
Show all reasoning and intermediate results leading to your answer.
One book of mathematical tables, such as Schaum's Mathematical Handbook,
may be used.
- 1.
- A graduate student will probably spent about 12.5 hours
of study outside class for each 3 credit hour class. But that
does not necessarily mean that you spent 12.5 hours a week
on Continuum Mechanics and 12.5 hours on Analysis in Mechanical
Engineering! Assume that your grade in Analysis can be computed as
| 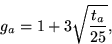 |
(1) |
where ta is the time you spent studying on Analysis each
week. So, you will get 1 grade point, a D, with no study and
4 grade points, an A, if you study 25 hours per week
on Analysis alone.
Also assume that your grade in Continuum Mechanics is
| 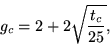 |
(2) |
where tc is the time you spent studing on Continuum Mechanics each
week. Find the maximum grade point average GPA,
| 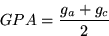 |
(3) |
that you can get if ta + tc = 25, i.e. if you study 25 hours on
Analysis and Continuum Mechanics combined. How much of the 25
hours would you spent on Analysis?
Solution.
- 2.
- Oblique shock waves in supersonic flows are described by
the ``shock polar"
| 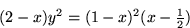 |
(4) |
Draw this curve as accurately as possible. Derive all symmetries,
intercepts, extents, asymptotes, and behavior at large |x|.
Solution.
- 3.
- Find the centroid of half a sphere by multiple integration.
Solution.



Up: Return
'Author: Leon van Dommelen'