EML 5060 Analysis in Mechanical Engineering 10/6/17
Closed book Van Dommelen 12:30-1:20 pm
Solutions should be fully derived showing all intermediate
results, using class procedures. Show all reasoning. Bare
answers are absolutely not acceptable, because I will assume they come
from your calculator (or the math handbook, sometimes,) instead of
from you. You must state what result answers what part of the
question. Answer what is asked; you do not get any credit for making
up your own questions and answering those. Use the stated procedures.
Give exact, fully simplified, answers where possible.
One book of mathematical tables, such as Schaum's Mathematical
Handbook, may be used, as well as a calculator, and a handwritten
letter-size formula sheet.
- Background: Graphical depiction of a function is often an
essential part to understand its properties.
Question: Analyze and very neatly graph
Discuss 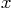 and
and 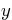 intercepts and extents, asymptotic behavior for
large positive
intercepts and extents, asymptotic behavior for
large positive 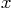 and large negative
and large negative 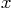 , horizontal, oblique and
vertical asymptotes, symmetries, local and global maxima and minima,
kinks, cusps, horizontal and vertical slopes and other
singularities. Given that there is only one inflection point in the
curve, where is it? And what is the concavity?
, horizontal, oblique and
vertical asymptotes, symmetries, local and global maxima and minima,
kinks, cusps, horizontal and vertical slopes and other
singularities. Given that there is only one inflection point in the
curve, where is it? And what is the concavity?
Draw the function very neatly, on suitably labelled axes, clearly
showing all features.
Solution.
- Background: There are various reasons you may want to
minimize the surface area of a barrel of given volume. (The barrel
is to be taken cylindrical, and the surface area to be taken to be
top and bottom circles plus curved surface.) One is to save
material cost. Another is to reduce heat conduction.
Question: Derive the ratio of height to diameter of the barrel
that has the smallest total surface area for a given volume.
Solution.
- Background: Areas of plates are important if you have an
axe to grind with paying for material costs.
Question: Derive the area of the region below 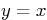 , above
, above
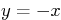 , and inside
, and inside 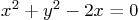 by using multiple integration with
those limits. Use polar coordinates
by using multiple integration with
those limits. Use polar coordinates 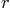 and
and 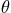 (with the same
origin) to do so. Lists the limits of integration, for both
(with the same
origin) to do so. Lists the limits of integration, for both 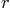 and
and
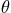 , if you do
, if you do 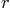 first and if you do
first and if you do 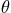 first. Lists
the reasons why one of the two options seems a lot better choice
than the other. Then integrate in the preferred way.
first. Lists
the reasons why one of the two options seems a lot better choice
than the other. Then integrate in the preferred way.
Solution.
![]() , above
, above
![]() , and inside
, and inside ![]() by using multiple integration with
those limits. Use polar coordinates
by using multiple integration with
those limits. Use polar coordinates ![]() and
and ![]() (with the same
origin) to do so. Lists the limits of integration, for both
(with the same
origin) to do so. Lists the limits of integration, for both ![]() and
and
![]() , if you do
, if you do ![]() first and if you do
first and if you do ![]() first. Lists
the reasons why one of the two options seems a lot better choice
than the other. Then integrate in the preferred way.
first. Lists
the reasons why one of the two options seems a lot better choice
than the other. Then integrate in the preferred way.