Up: Return
EML 5060 Analysis in Mechanical Engineering 11/7/03
Closed book Van Dommelen 9:15-10:05 pm
Solutions of the ODE should be fully derived using class procedures:
be sure to show your complete derivation, not just the answer. ``My
calculator / mathematical handbook says that this is the solution'' is
not acceptable. Clean up your answer as much as possible, using class
procedures. One book of mathematical tables, such as Schaum's
Mathematical Handbook, may be used, as well as a calculator, and
a handrwitten letter-size formula sheet.
- 1.
- The streamlines of a linearly varying velocity field
satisfy the ordinary differential equation:
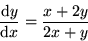
Find these streamlines.
Solution.
- 2.
- Solve the following equation of motion for a spring mass system
in an exponentially decreasing resonance condition:
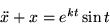
where k is a negative constant. The particular solution can be
guessed. Why would the solution not apply for k=0, k=2i, and
k=-2i?
Solution.
- 3.
- The particle motion in the velocity field of question 1 is more
fully described by the first order system of equations
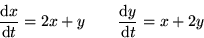
Solve this using the class procedure for systems. Sketch
the motion in the (x,y) plane from
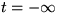 (where is the particle then?)
to
(where is the particle then?)
to 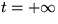 (where is the particle then?). Compare with the
streamline shape from question 1.
(where is the particle then?). Compare with the
streamline shape from question 1.
Solution.
Up: Return
![]()
![]()
![]()
![]()
![]()
![]()